What are concrete resources?

You’ll probably know them as place value counters, numicon, dienes etc. – these are all examples of concrete resources.

Concrete resources (also referred to as manipulatives) are objects or physical resources that children can handle and manipulate to aid their understanding of different maths concepts. A mastery teaching approach encourages children of all ages to keep using these concrete resources, in Key Stage 1 (KS1) as well as Key Stage 2 (KS2). While the abstract nature of maths can be confusing for children, through the use of these concrete, practical resources, they are able to ‘see’ the maths and make sense of what is actually happening.

Using the Concrete-Pictorial-Abstract (CPA) approach 

Once children are confident with a concept using concrete resources, they progress to drawing pictorial representations or quick sketches of the objects. By doing this, they are no longer manipulating the physical resources, but are still benefiting from the visual support the resources provide.

Once children have a secure understanding of the concept through the use of concrete resources and visual images, they are then able to move on to the abstract.

Taking short division as an example, the formal method is difficult to understand. Although children can be taught the method without understanding the maths behind it, this can lead to confusion errors and difficulties with retaining or remembering the skills or knowledge required to complete division calculations successfully. If children are taught to use place value counters (concrete) they can make sense of the method. Once confident with this, they can move on to recording the place value counters pictorially and eventually move on to the abstract formal written method. 

Why these teaching aids are a crucial part of mastery teaching?

In the past, children were taught procedures, but not why or how the procedure worked. In other words, children learnt the methods to get to an answer, without any understanding of the maths behind each method or procedure. While there are children who are able to access the maths through just learning a procedure by rote, many others have great difficulty coping with the abstract nature of it. Teaching methods without meaning leads to misconceptions, errors and difficulties in retaining the methods. Once children can actually ‘see’ the maths, they are much more likely to understand and accurately remember the methods. 

Using concrete resources at home

Place value:

​​

Home alternatives for learning about place value

Straws, lollipop sticks or anything else that can be bundled together are a fantastic alternative to Dienes equipment. For example, individual straws can represent the ones and bundled together into tens to represent the tens. Lego is another good alternative, using individual bricks for the ones and joining together to make tens. The children are able to visually see the link between the size of the individual straws/bricks and the bundles of ten straws/bricks. Downloading and printing images of Numicon onto card is a free alternative to buying a set of Numicon. Place value counters can easily be made using plain counters and a marker pen. Alternatively, you use money, with 1p representing the ones, 10p then tens and £1 the hundreds.

Written calculation methods

Once children are secure with place value, they are able to use the Dienes equipment or Numicon as a first step in understanding the written methods for column addition and subtraction, grid method multiplication and bus stop/short division. 

The equipment is set out in columns on a baseboard as a concrete representation of the formal written method. Children use it to add/subtract the ones first and then the tens. If the calculation requires exchanging, they are able to physically exchange the ten ones for a ten and vice versa.  

As with place value, children progress to replacing Dienes equipment or Numicon with place value counters to solve calculations involving the four operations.

 

 

 

 

 

 

 

Fractions:

Fractions are an area of maths children can find particularly difficult, due to their abstract nature. For this reason, it is essential they have practical resources to hold and manipulate or play with. Fraction cubes or circles and Numicon are very useful for aiding understanding of the fraction of a whole, add to that using place value counters, multi-link cubes, skittles or smarties and you can demonstrate a real range of fraction concepts: from the basics of what a fraction is; to recognising equivalent fractions; understanding what happens when a fraction is greater than one and for adding/subtracting fractions.

Finding fractions of amounts is another concept children can have difficulty grasping, particularly when the numerator is greater than one. Using resources, such as cubes or counters – or beans, skittles, smarties or pasta pieces – and physically sharing them out really helps children understand.

Here is a video showing fractions of amounts using the pictorial example of the bar model to explain how it can be used to help children understand.

Fractions of amounts using the bar model

Home alternatives for learning about fractions 

There are lots of cheap/free alternatives to buying fraction resources. Fraction circles can be made out of paper plates, or downloaded off the internet and printed onto coloured card.

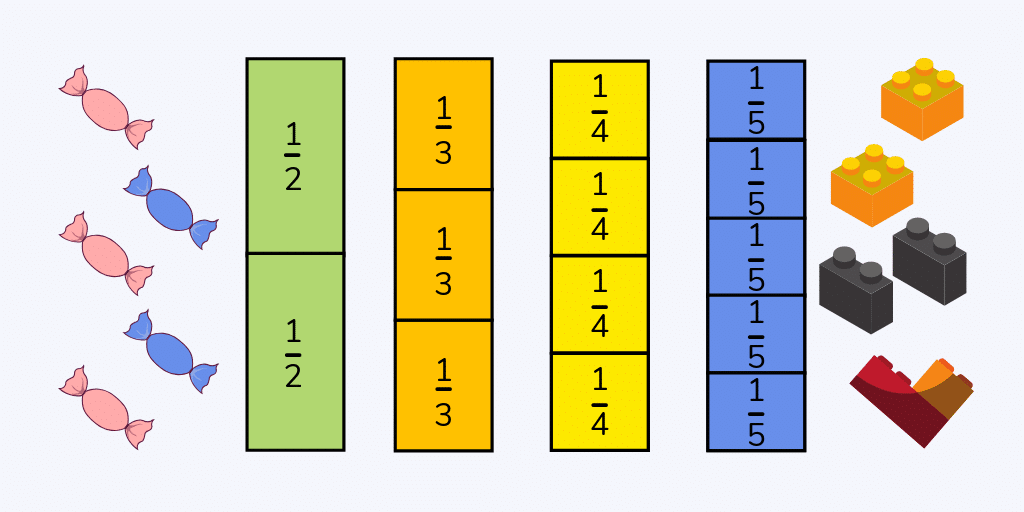
Lego and printable fraction strips are a good alternative to buying fraction cubes. For fractions of amounts, anything that can be shared out can be used. Food works particularly well for this. For example: raisins, grapes, sweets etc.

 

Adding fractions with the same denominator